Are you looking for an answer to the topic “What is functionally complete set of connectives Mcq?“? We answer all your questions at the website Ecurrencythailand.com in category: +15 Marketing Blog Post Ideas And Topics For You. You will find the answer right below.
In the logic gate, a functionally complete collection of logical connectives or Boolean operators is one which can be used to express all possible truth tables by combining members of the set into a Boolean expression.Since every formula is obtained starting with propositional variables and then repeatedly applying connectives, this shows the theorem. Our next theorem uses this technique to show that the set {¬, ↔} is not functionally complete. Theorem 2.7. The set {¬, ↔} is not functionally complete.The set (AND, OR, NOT) is a functionally complete set.

Is ↔ a complete set of connectives?
Since every formula is obtained starting with propositional variables and then repeatedly applying connectives, this shows the theorem. Our next theorem uses this technique to show that the set {¬, ↔} is not functionally complete. Theorem 2.7. The set {¬, ↔} is not functionally complete.
Is the set or and functionally complete?
The set (AND, OR, NOT) is a functionally complete set.
Prove a Set of Connectives Is Functionally Complete
Images related to the topicProve a Set of Connectives Is Functionally Complete

Which of the following sets of connective is not functional complete?
The EX-NOR is not functionally complete because we cannot synthesize all Boolean functions using EX-NOR gate only.
Which of the following is functionally complete set A and or B and XOR C not or D and/or not?
1 Answer. C) obviously functionally complete.
What is functionally complete set of connectives?
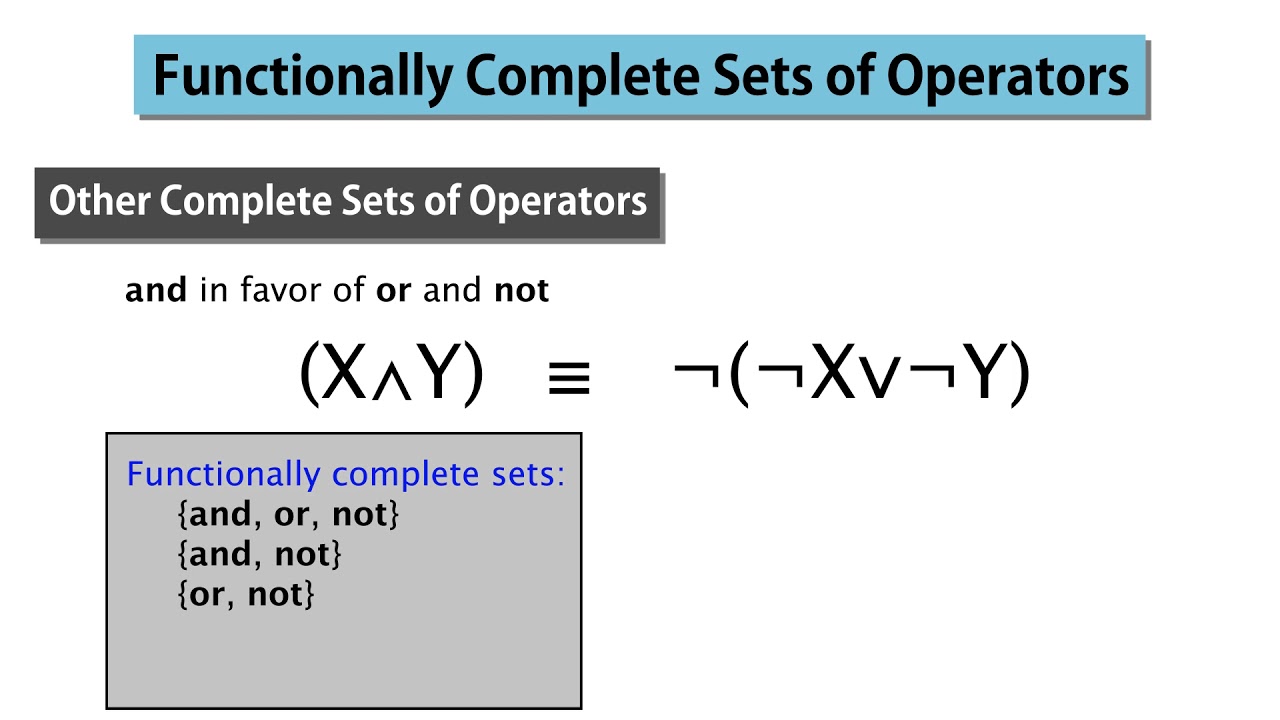
In logic, a functionally complete set of logical connectives or Boolean operators is one which can be used to express all possible truth tables by combining members of the set into a Boolean expression. A well-known complete set of connectives is { AND, NOT }, consisting of binary conjunction and negation.
How do you show that a set of connectives is functionally complete?
- • A set of logical connectives is called functionally. complete if every boolean expression is equivalent to one involving only these connectives.
- • The set {¬,∨,∧} is functionally complete. …
- • The sets {¬,∨} and {¬,∧} are functionally complete.
Which is are functionally complete?
A set of operations is said to be functionally complete or universal if and only if every switching function can be expressed by means of operations in it.
See some more details on the topic What is functionally complete set of connectives Mcq? here:
Functional completeness – Wikipedia
In logic, a functionally complete set of logical connectives or Boolean operators is one which can be …
Which of the following is functionally complete set? – Toppr
In logic, a functionally complete set of logical connectives or Boolean operators is one which can be used to express all possible truth tables by combining …
Functional Completeness in Digital Logic – GeeksforGeeks
A set of operations is said to be functionally complete or universal if and only if every switching function can be expressed by means of …
Boolean Functions Questions and Answers – Sanfoundry
This set of Discrete Mathematics Multiple Choice Questions & Answers (MCQs) … The set for which the Boolean function is functionally complete is ______
Is and or truth functionally complete?
…
Truth-functional Completeness.
| A | B | (A & B) v (~A & B) |
|---|---|---|
| T | F | F |
| F | T | T |
| F | F | F |
How do you prove a set of connectives is not functionally complete?
To prove that a set C of connectives is not complete, we start by finding a property (expressed as a pred- icate) that every formula that uoc C has, but not every formula in general. Then we prove that every formula that uoc C has the desired property.
Are NAND gates functionally complete?
The NAND and NOR operators are each functionally complete. That is, NAND and NOR are Sheffer operators.
1 Functionally Complete Sets of Operators FINAL
Images related to the topic1 Functionally Complete Sets of Operators FINAL
Are NOR gates functionally complete?
NOR is a functionally complete operation—NOR gates can be combined to generate any other logical function.
Are XOR gates functionally complete?
NOR and NAND are the only functionally complete singleton gate sets. Hence, XOR is not functionally complete on its own (or together with NOT, since as point out above NOT can be created using XOR). XOR can be complemented to a two-element functionally complete gate sets.
Which of the following logical operator S is are functionally complete?
Which of the following logical operator(s) is/are functionally complete? Explanation: All the three logical operators given are functionally complete because all other logical operators can be implemented using these three.
Is multiplexer functionally complete?
2. 2-1 multiplexer is functionally complete provided we have external 1 and 0 available. For NOT gate, use x as select line and use 0 and 1 as inputs.
What does it mean to be truth functionally complete?
1. A set of truth-functional operators is said to be truth-functionally complete (or expressively adequate) just in case one can take any truth-function whatsoever, and construct a formula using only operators from that set, which represents that truth-function.
What is DNF in Boolean algebra?
In boolean logic, a disjunctive normal form (DNF) is a canonical normal form of a logical formula consisting of a disjunction of conjunctions; it can also be described as an OR of ANDs, a sum of products, or (in philosophical logic) a cluster concept.
Is implication and negation functionally complete?
Disjunction plus negation as well as conjunction combined with negation are functionally complete. Hence, implication combined with a false constant is also functionally complete.
How do you prove a set is complete?
We must show that a∈b. Assume that ‖a−x0‖>R then ‖a−x0‖=R+k where k>0. We have ‖a−x0‖≤‖a−bn‖+‖bn−x0‖≤‖a−bn‖+R but this is a contradiction since we can find an N such that ‖a−bN‖<ϵ=k since bn→a. Q.E.D.
What is adequate set of connectives?
If a set of connectives is sufficient to express every possible propositional formula, we call it an adequate set of connectives. That is, any other connective not in this set is definable in terms of the ones in this set. Theorem 1. {∧,∨,¬} is an adequate set of connectives.
Mathematical Logic : Functionally Complete Set of Connectives
Images related to the topicMathematical Logic : Functionally Complete Set of Connectives
What are logic gates?
A logic gate is a device that acts as a building block for digital circuits. They perform basic logical functions that are fundamental to digital circuits. Most electronic devices we use today will have some form of logic gates in them.
Is used to implement the Boolean function Mcq?
_________ is used to implement the Boolean functions. Explanation: To implement a Boolean function logic gates are used.
Related searches to What is functionally complete set of connectives Mcq?
- prove that the set is not functionally complete
- show that is a functionally complete set of connectives
- is xor functionally complete
- what is functionally complete set of connectives mcq questions
- what is functionally complete set of connectives mcq answers
- are muxes functionally complete
- the functionally complete set is mcq
- which of the following is functionally complete set
- what is functionally complete set of connectives mcq part b
- are xor gates functionally complete
- what is functionally complete set of connectives mcq questions and answers
- what is functionally complete set of connectives mcq quizlet
- show is a complete set of connectives
Information related to the topic What is functionally complete set of connectives Mcq?
Here are the search results of the thread What is functionally complete set of connectives Mcq? from Bing. You can read more if you want.
You have just come across an article on the topic What is functionally complete set of connectives Mcq?. If you found this article useful, please share it. Thank you very much.