Are you looking for an answer to the topic “What is Green theorem in calculus?“? We answer all your questions at the website Ecurrencythailand.com in category: +15 Marketing Blog Post Ideas And Topics For You. You will find the answer right below.
In vector calculus, Green’s theorem relates a line integral around a simple closed curve C to a double integral over the plane region D bounded by C. It is the two-dimensional special case of Stokes’ theorem.Green’s theorem gives a relationship between the line integral of a two-dimensional vector field over a closed path in the plane and the double integral over the region it encloses. The fact that the integral of a (two-dimensional) conservative field over a closed path is zero is a special case of Green’s theorem.Put simply, Green’s theorem relates a line integral around a simply closed plane curve C and a double integral over the region enclosed by C. The theorem is useful because it allows us to translate difficult line integrals into more simple double integrals, or difficult double integrals into more simple line integrals.
What is the meaning of Green theorem?
Green’s theorem gives a relationship between the line integral of a two-dimensional vector field over a closed path in the plane and the double integral over the region it encloses. The fact that the integral of a (two-dimensional) conservative field over a closed path is zero is a special case of Green’s theorem.
Why do we use Green’s theorem?
Put simply, Green’s theorem relates a line integral around a simply closed plane curve C and a double integral over the region enclosed by C. The theorem is useful because it allows us to translate difficult line integrals into more simple double integrals, or difficult double integrals into more simple line integrals.
Green’s Theorem
Images related to the topicGreen’s Theorem

What is the Green’s theorem and explanation with 3 example?
The first piece is the half circle, oriented from right to left (labeled C1 and in blue, below). The second piece is the line segment, oriented from left to right (labeled C2 and in green). and (let u=cost, du=−sintdt) ∫π0sintcos2tdt=∫−11−u2du=−u33|−11=−(−1)/3+1/3=2/3. Therefore.
What is green Gauss theorem?
Green Gauss Theorem relates the volume & surface integrals. The most common form of Gauss’s theorem is the Gauss divergence theorem which you have studied in previous courses. This Gauss Divergence theorem can also be represented using index notations.
Who discovered Green theorem?
In mathematics, Green’s identities are a set of three identities in vector calculus relating the bulk with the boundary of a region on which differential operators act. They are named after the mathematician George Green, who discovered Green’s theorem.
Where is Green’s theorem used in real life?
Identities derived from Green’s theorem like above play a key role in reciprocity in electromagnetism, the entry in wikipedia has a lot of examples. Some real life applications include using the reciprocity to evaluate the excitation from an impulse in waveguide or antenna designs.
What are the two forms of Green’s theorem?
Green’s theorem has two forms: a circulation form and a flux form, both of which require region D in the double integral to be simply connected. However, we will extend Green’s theorem to regions that are not simply connected.
See some more details on the topic What is Green theorem in calculus? here:
Calculus III – Green’s Theorem – Pauls Online Math Notes
Section 5-7 : Green’s Theorem. In this section we are going to investigate the relationship between certain kinds of line integrals (on …
Green’s theorem (article) | Khan Academy
Green’s theorem is one of four major theorems at the culmination of multivariable calculus: Green’s theorem; 2D divergence theorem; Stokes’ theorem …
The idea behind Green’s theorem – Math Insight
Green’s theorem is simply a relationship between the macroscopic circulation around the curve C and the sum of all the microscopic circulation that is inside C.
Green’s Theorem – Calculus Volume 3 – BC Open Textbooks
Put simply, Green’s theorem relates a line integral around a simply closed plane curve C and a double integral over the region enclosed by C. The theorem is …
What is the difference between Green’s theorem and stock theorem?
Stokes’ theorem is basically a more general green’s theorem where the surface is not restricted to the xy plane. In this case you dot the curl of your vector field with the normal vector to your surface instead of the k unit vector.
Green’s theorem example 1 | Multivariable Calculus | Khan Academy
Images related to the topicGreen’s theorem example 1 | Multivariable Calculus | Khan Academy

How do you read Green theorem?
Green’s theorem is simply a relationship between the macroscopic circulation around the curve C and the sum of all the microscopic circulation that is inside C. If C is a simple closed curve in the plane (remember, we are talking about two dimensions), then it surrounds some region D (shown in red) in the plane.
When can you apply Greens Theorem?
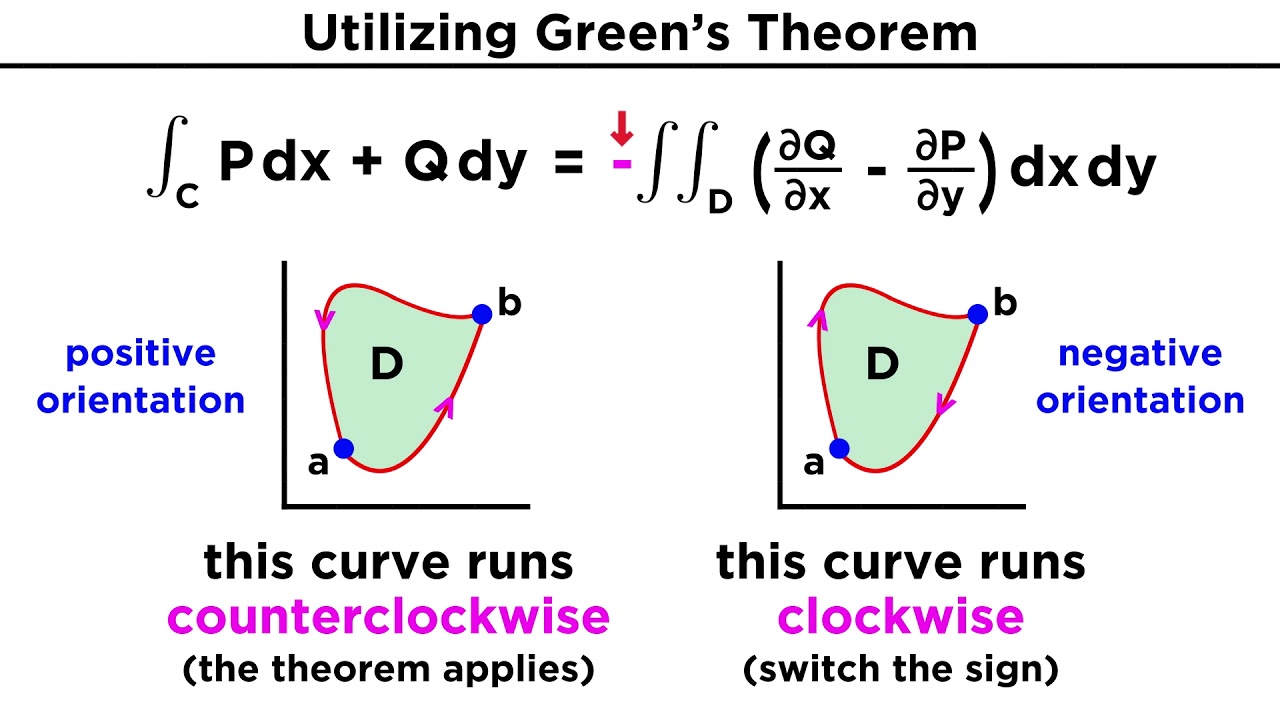
Warning: Green’s theorem only applies to curves that are oriented counterclockwise. If you are integrating clockwise around a curve and wish to apply Green’s theorem, you must flip the sign of your result at some point.
Who is Green’s theorem named after?
history of mathematics
The Gauss-Green-Stokes theorem, named after Gauss and two leading English applied mathematicians of the 19th century (George Stokes and George Green), generalizes the fundamental theorem of the calculus to functions of several variables.…
What is green formula?
The Green formulas are obtained by integration by parts of integrals of the divergence of a vector field that is continuous in ¯D=D+Γ and that is continuously differentiable in D.
Why We Use Del operator?
The del operator (∇) is an operator commonly used in vector calculus to find derivatives in higher dimensions. When applied to a function of one independent variable, it yields the derivative. For multidimensional scalar functions, it yields the gradient.
What is circulation and flux?
Flux measures the rate that a field crosses a given line; circulation measures the tendency of a field to move in the same direction as a given closed curve.
Green’s Theorem, explained visually
Images related to the topicGreen’s Theorem, explained visually

What is curl vector field?
In vector calculus, the curl is a vector operator that describes the infinitesimal circulation of a vector field in three-dimensional Euclidean space. The curl at a point in the field is represented by a vector whose length and direction denote the magnitude and axis of the maximum circulation.
Which of the following is not an application of Green’s theorem?
Volume of plane figures is not an application of greens theorem.
Related searches to What is Green theorem in calculus?
- what is green theorem in calculus
- find area using greens theorem
- use greens theorem to evaluate the line integral
- stokes theorem
- green’s theorem mathematics
- where c is the triangular curve with vertices
- Find area using green’s theorem
- greens theorem
- Green’s theorem calculator
- what does green’s theorem find
- using green’s theorem
- Stokes’ theorem
- Divergence theorem
- divergence theorem
- Green’s theorem
- what is de moivre’s theorem
- greens theorem reverse
- the function in green’s theorem will be
- explain green’s theorem
- greens theorem calculator
- Green’s theorem reverse
- green’s theorem in electrodynamics
Information related to the topic What is Green theorem in calculus?
Here are the search results of the thread What is Green theorem in calculus? from Bing. You can read more if you want.
You have just come across an article on the topic What is Green theorem in calculus?. If you found this article useful, please share it. Thank you very much.