Are you looking for an answer to the topic “What is harmonic conjugate in complex analysis?“? We answer all your questions at the website Ecurrencythailand.com in category: +15 Marketing Blog Post Ideas And Topics For You. You will find the answer right below.
The harmonic conjugate to a given function is a function such that. is complex differentiable (i.e., satisfies the Cauchy-Riemann equations). It is given by. where , , and. is a constant of integration.Definition of harmonic conjugates
: the two points that divide a line segment internally and externally in the same ratio.In Complex Analysis, Harmonic Conjugate are those which satisfy both Cauchy–Riemann equations & Laplace’s equation . The Cauchy–Riemann equations on a pair of real-valued functions of two real variables u(x,y) and v(x,y) are the two equations: Now, Thus, which is the Laplace Equation.

What is meant by harmonic conjugate?
Definition of harmonic conjugates
: the two points that divide a line segment internally and externally in the same ratio.
What is the meaning of harmonic conjugate in complex analysis?
In Complex Analysis, Harmonic Conjugate are those which satisfy both Cauchy–Riemann equations & Laplace’s equation . The Cauchy–Riemann equations on a pair of real-valued functions of two real variables u(x,y) and v(x,y) are the two equations: Now, Thus, which is the Laplace Equation.
How to Find a Harmonic Conjugate for a Complex Valued Function
Images related to the topicHow to Find a Harmonic Conjugate for a Complex Valued Function

What is harmonic conjugate in complex numbers?
If the real part of a complex function is harmonic, then there exists a harmonic imaginary part, so that the function is analytic. The imaginary part is known as the harmonic conjugate of the real part.
How do you find the harmonic conjugate?
We can obtain a harmonic conjugate by using the Cauchy Riemann equations. ∂v ∂y = 2x + g/(y) = ∂u ∂x =3+2x – 4y. where C is a constant. To satisfy v(0,0) = 0 we need v(0,0) = g(0) = C = 0 and thus v(x, y) = x + 2xy + 2×2 + 3y – 2y2.
What is the formula for harmonic conjugate?
Since u is harmonic (on the simply connected domain C), there has to be a harmonic conjugate v. Let F=u+iv be the corresponding holomorphic function. It follows from (the derivation of) Cauchy-Riemann’s equations that: F′=u′x−iu′y=−12xy+8x−7y+3+i(6×2+7x−6y2+8y−4).
What is the meaning of harmonic function?
harmonic function, mathematical function of two variables having the property that its value at any point is equal to the average of its values along any circle around that point, provided the function is defined within the circle.
What is harmonic conjugate of a point?
Harmonic conjugate: The points PQ which divides the line segment AB in the same ratio m : n internally and externally then P and Q are said to be harmonic conjugates of each other wrt A and B. Formula: If point P divides AB in the ratio m : n internally then harmonic conjugate divides AB in the ratio (-m : n).
See some more details on the topic What is harmonic conjugate in complex analysis? here:
Harmonic conjugate – Wikipedia
For geometric conjugate points, see Projective harmonic conjugate. “Conjugate function” redirects here. For the convex conjugate of a function, …
Harmonic Conjugate – Concept and Solved Examples
If the real part of a complex function is harmonic, then there exists a harmonic imaginary part, so that the function is analytic.
What is harmonic conjugate? – Quora
In Complex Analysis, Harmonic Conjugate are those which satisfy both Cauchy–Riemann equations & Laplace’s equation . The Cauchy–Riemann equations on a pair …
Calculating a harmonic conjugate – Math Stack Exchange
Yet another shortcut. Since u is harmonic (on the simply connected domain C), there has to be a harmonic conjugate v. Let F=u+iv be the corresponding …
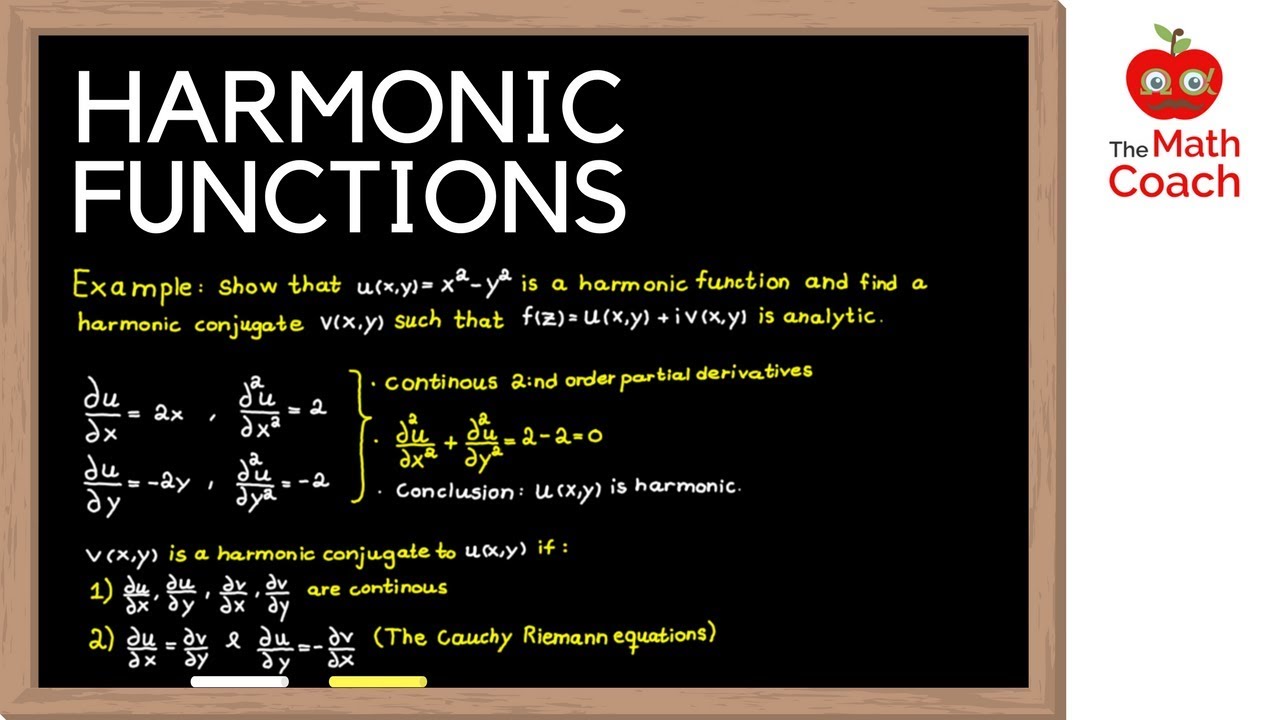
Harmonic functions | Harmonic conjugate | Complex Analysis #3
Images related to the topicHarmonic functions | Harmonic conjugate | Complex Analysis #3
What is the conjugate of a function?
1. The conjugate function f* (y) is the maximum gap between the linear function yx and the function f (x). Example 1 (Affine function) f (x) = ax + b. By definition, the conjugate function is given by f∗ (y) = supx (yx − ax − b).
Are all analytic functions harmonic?
Not all harmonic functions are complex analytic because satisfying the Cauchy-Riemann equations is stronger than satisfying Laplace’s equation. For example, there exist purely real nonconstant functions which are harmonic on the whole plane, whereas no such function can be complex analytic.
Are harmonic conjugates unique?
Thus w = v + a, for some complex number a. Thus harmonic conjugates are unique up to adding a constant. Example 13.4. Show that u = xy is harmonic on the whole complex plane and find a harmonic conjugate.
How do you show a harmonic function?
So a function is harmonic if it satisfies Laplace’s equation. The operator ∇2 is called the Laplacian and ∇2u is called the Laplacian of u. (v) div gradu = v · vu = ∇2u = uxx + uyy (vi) curl gradu = v × vu = 0 (vii) div curlF = v · v × F = 0. The connection between analytic and harmonic functions is very strong.
What is harmonic progression in maths?
In mathematics, a harmonic progression (or harmonic sequence) is a progression formed by taking the reciprocals of an arithmetic progression.
How to find a Harmonic Conjugate Complex Analysis
Images related to the topicHow to find a Harmonic Conjugate Complex Analysis
Why are harmonic functions called harmonic?
The descriptor “harmonic” in the name harmonic function originates from a point on a taut string which is undergoing harmonic motion. The solution to the differential equation for this type of motion can be written in terms of sines and cosines, functions which are thus referred to as harmonics.
Why are harmonic functions important?
Harmonic functions are called potential functions in physics and engineering. Potential functions are extremely useful, for example, in electromagnetism, where they reduce the study of a 3-component vector field to a 1-component scalar function.
Related searches to What is harmonic conjugate in complex analysis?
- harmonic in complex analysis
- complex numbers conjugate
- harmonic function
- harmonic conjugate function complex analysis
- harmonic conjugate example
- Harmonic function
- how to find harmonic conjugate of a complex function
- harmonic conjugate of y/(x^2+y^2)
- harmonic conjugate of a complex function
- harmonic conjugate examples pdf
- harmonic conjugate function calculator
- construction of harmonic conjugate
- complex conjugate of harmonic functions
- harmonic conjugate finder
- what is harmonic conjugate in complex analysis
- harmonic function in complex analysis examples
Information related to the topic What is harmonic conjugate in complex analysis?
Here are the search results of the thread What is harmonic conjugate in complex analysis? from Bing. You can read more if you want.
You have just come across an article on the topic What is harmonic conjugate in complex analysis?. If you found this article useful, please share it. Thank you very much.